Brittle Damage Package in Abaqus- Johnson-Holmquis and Beissel Model

Introduction to Brittle Damage Modeling in Abaqus: Johnson-Holmquist and Beissel Models

Brittle damage refers to the failure mechanism observed in brittle materials such as concrete, glass, ice, and ceramics. Unlike ductile materials, which undergo significant plastic deformation before failure, brittle materials typically fail suddenly with little to no warning and minimal plastic deformation. Here’s a detailed explanation of brittle damage in these materials

Brittle materials, such as ceramics, concrete, glass, and ice, exhibit unique failure characteristics under high-stress conditions, including sudden fracture with minimal plastic deformation. To accurately simulate the behavior of these materials under dynamic loading (e.g., impact, blast, or high-velocity events), advanced constitutive models are required. In Abaqus, a finite element analysis (FEA) software, the Johnson-Holmquist (JH) and Beissel models are widely used to model brittle damage and failure. These models are particularly suited for high-strain-rate applications where traditional elastic-plastic models are insufficient

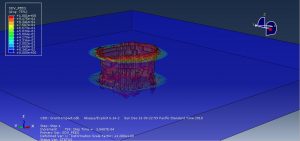
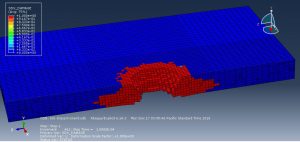
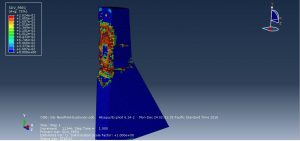
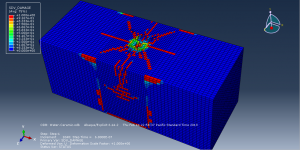
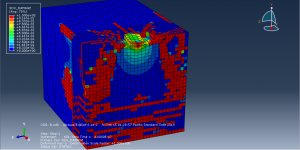
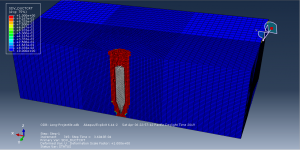
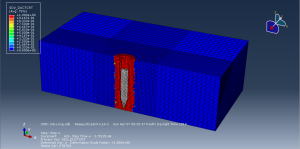
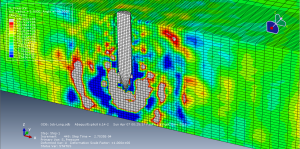
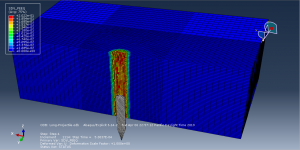
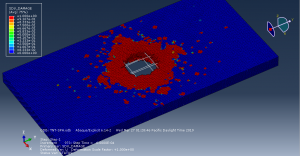
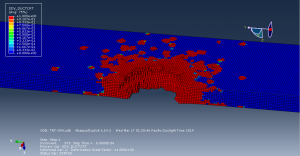
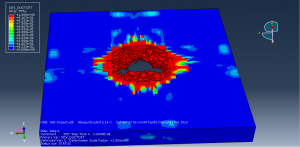
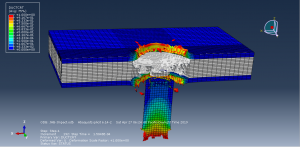
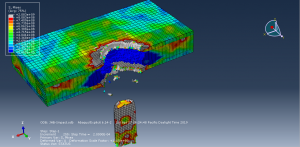
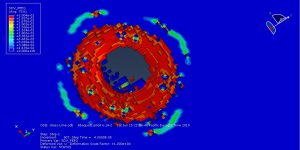
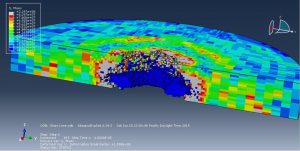
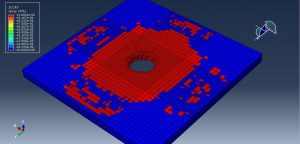
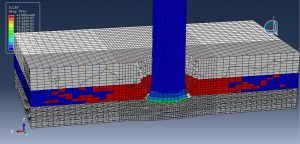
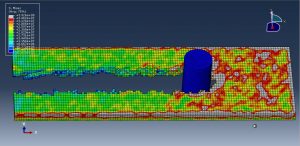
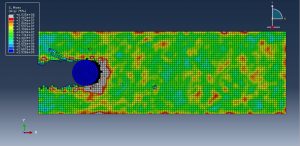
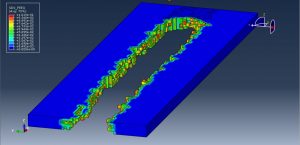
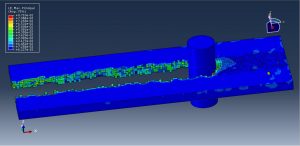
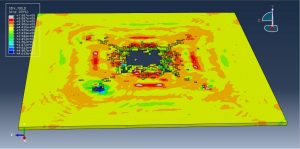
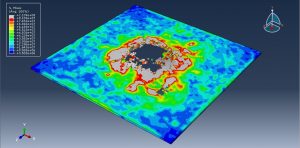
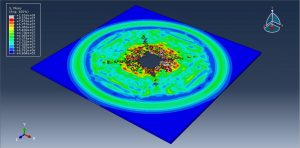
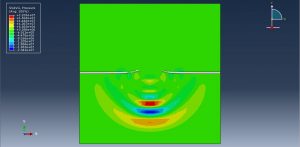
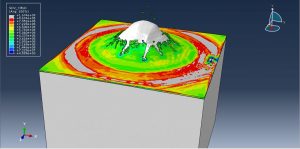
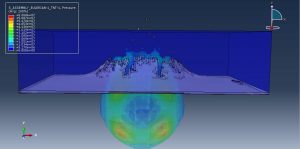
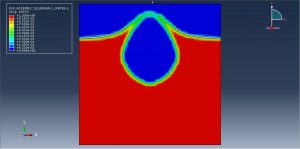
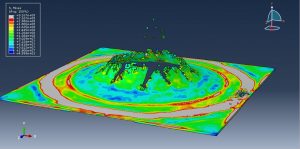
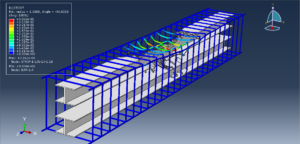
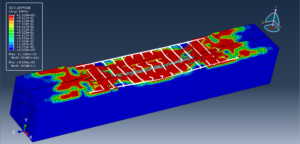
Characteristics of Brittle Materials

Low Tensile Strength: Brittle materials are generally much stronger in compression than in tension. They tend to fail under tensile stress at much lower levels compared to compressive stress

Linear Elastic Behavior: Up to the point of failure, brittle materials usually exhibit linear elastic behavior, meaning they deform proportionally to the applied stress and return to their original shape when the stress is removed

Lack of Plastic Deformation: Brittle materials do not undergo significant plastic deformation Instead, they fracture abruptly once the stress exceeds their strength

Mechanisms of Brittle Damage

Microcrack Formation: Brittle damage often initiates at the microscopic level with the formation of microcracks. These micro-cracks can be inherent flaws within the material or can develop due to applied stress

Stress Concentration: Microcracks and other defects (like voids or inclusions) act as stress Stress Concentration: Microcracks and other defects (like voids or inclusions) act as stress

Crack Propagation: Once a critical stress level is reached, the microcracks begin to grow and coalesce. This process is often rapid and can lead to catastrophic failure. The propagation of cracks is typically governed by the principles of fracture mechanics

Fracture: The final stage of brittle damage is a fracture, where the material separates into two or more pieces. This can occur along specific planes of weakness, such as grain boundaries in polycrystalline materials

Johnson-Holmquist (JH) Models

The Johnson-Holmquist family of models is specifically designed for brittle materials subjected to high-pressure and high-strain-rate conditions. These models account for the material’s strength, damage, and failure under dynamic loading. There are two primary versions of the JH model

Johnson-Holmquist Ceramic Model (JH-I and JH-II)

JH-I: This model assumes that the material’s strength is independent of damage until failure. It uses a polynomial equation of state (EOS) to describe the pressure-volume relationship and a strength model that depends on pressure, strain rate, and damage

JH-II: This model incorporates a more sophisticated damage evolution law, where the material’s strength degrades as damage accumulates. It is more suitable for materials that exhibit gradual softening under load

Key Features

Strength Model: Describes the material’s yield strength as a function of pressure, strain rate, and damage

Damage Model: Tracks the accumulation of damage based on plastic strain and pressure

Equation of State (EOS): Defines the relationship between pressure, volume, and internal energy

Failure Criterion: The material fails when the damage reaches a critical value

Applications

Impact and penetration simulations (e.g., ballistic impact on ceramic armor)

High-velocity collisions involving brittle materials

Johnson-Holmquist Concrete Model (JH-C)

This variant is tailored for concrete and similar materials. It includes modifications to account for the unique behavior of concrete under high-pressure and high-strain-rate conditions, such as compaction and pore collapse

Key Features

Pressure-Dependent Strength: Captures the increase in strength under high confinement

Damage Evolution: Tracks damage accumulation due to tensile and compressive stresses

Strain Rate Effects: Accounts for the increase in material strength at higher strain rates

Applications

Blast and impact simulations on concrete structures

Dynamic loading of reinforced concrete

 

 Beissel Model

The Beissel model is another constitutive model used in Abaqus for simulating brittle materials, particularly under high-strain-rate conditions. It is an extension of the Johnson-Holmquist model and is often used for ceramics and glass

Key Features

Strength and Damage Coupling: The model couples the material’s strength with damage evolution, allowing for a more accurate representation of softening behavior

Pressure Sensitivity: Accounts for the influence of hydrostatic pressure on material strength

Strain Rate Effects: Includes strain rate dependence to capture dynamic loading effects

Failure Criterion: Defines failure based on accumulated damage or critical stress/strain thresholds

Applications

High-velocity impact simulations (e.g., projectile impact on glass or ceramic targets)

Dynamic fracture analysis of brittle materials

Implementation in Abaqus

In Abaqus, both the Johnson-Holmquist and Beissel models are implemented as user-defined material models (UMAT or VUMAT for explicit dynamics)

Examples of Brittle Damage

Concrete: In concrete, brittle damage can manifest as cracking due to tensile stresses, shrinkage, or thermal expansion. Over time, these cracks can propagate and lead to structural failure

Glass: Glass typically fails by the propagation of surface flaws under tensile stress, leading to sudden and often catastrophic fracture

Ice: Ice can fracture under stress, especially at low temperatures. This is commonly observed in glaciers and ice sheets

Ceramics: Ceramics are prone to brittle failure due to their crystalline structure and the presence of microcracks. They often fail under impact or thermal shock

 Advantages of Using JH and Beissel Models in Abaqus

Accurate Brittle Failure Prediction: These models capture the sudden and catastrophic failure of brittle materials under dynamic loading

High-Strain-Rate Capability: Suitable for simulations involving impact, blast, and high-velocity events

Damage Evolution Tracking: Provides insights into how damage initiates and propagates in the material

Versatility: Can be applied to a wide range of brittle materials, including ceramics, concrete, glass, and ice